Foundations
Clock waveforms can be represented as periodic trains of trapezoid-shaped pulses as shown in Figure 1.
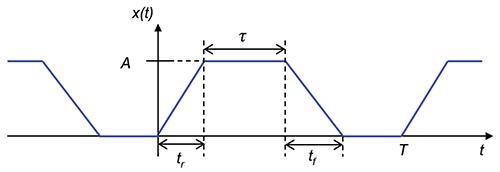
Each pulse is described by the key parameters: period T (and thus the fundamental frequency), amplitude A, rise time tr, fall time tf, and the on-time or pulse width τ. In this article, we investigate the effect of these pulse parameters on the spectrum of the clock waveform.
When the rise and fall times are equal, the one-sided Fourier spectrum of the trapezoidal clock signal can be represented as
![]() (1)
(1)
where the magnitudes of the (complex) Fourier coefficients are given by
![]() (2)
(2)
 (3)
(3)
Note that when the duty cycle of the signal is 50%, that is, , the first sine term in Equation 3 becomes
 (4)
(4)
which is zero for even n. Thus, there are no even harmonics when the duty cycle is 50% (which is a reasonable assumption for the clock signals).
The spectral coefficients in Equation (3) exist only at the discrete frequencies . The continuous envelope of these spectral components is obtained by replacing in Equation (3):
![]() (5)
(5)
or in dB,
 (6)
(6)
The (envelope) bounds in dB are shown in Figure 2.
Before we investigate the effects of various parameters on the frequency content of the clock signals, an extremely important observation can be made from the bounds shown in Figure 2.
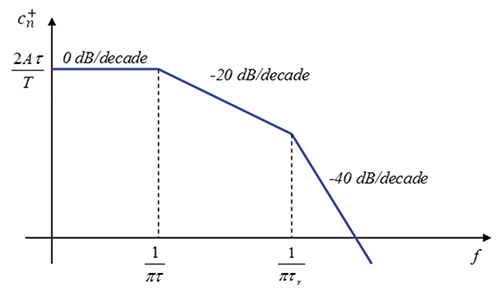
Note that above the frequency the amplitudes of the spectral components are attenuated at a rate of 40 dB/decade. It seems reasonable, therefore, to postulate that somewhere beyond this frequency these amplitudes are negligible (as compared to the magnitudes of the components at lower frequencies) and can be neglected in the Fourier series expansion shown in Equation 1.
A reasonable choice for that frequency is
![]() (7)
(7)
This is the origin of one of the EMC rules which states that the bandwidth (BW) of a trapezoidal signal (the highest significant frequency) is
![]() (8)
(8)
Thus the pulses having short rise/fall times have larger high-frequency content that do pulses with long rise/fall times.
In the next section we will verify this statement, show the frequency content of a clock signal with 50% duty cycle (no even harmonics), and investigate the effect of various pulse parameters on the frequency spectrum.
Verification
The experimental setup for spectral measurements is shown in Figure 3.

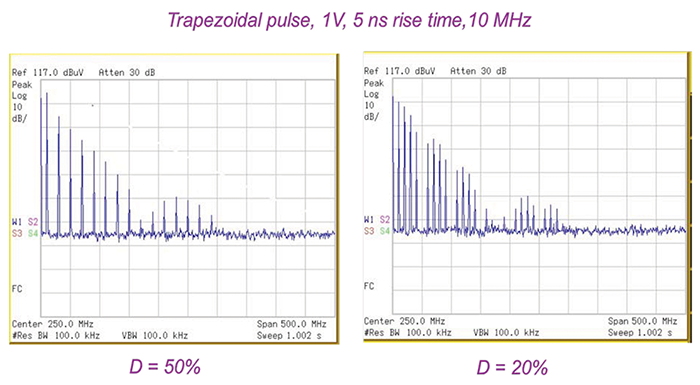
Figure 4 shows the frequency spectrum of a 1V trapezoidal pulse, with a fundamental frequency of 10 MHz and 5 ns risetime and two different duty cycles.
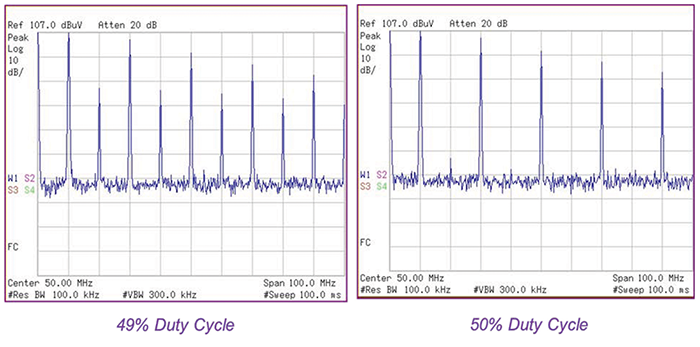
Note that the 49% duty cycle signal contains both the odd and even harmonics while the 50% duty cycle signal contains the odd harmonics only.
Figure 5 shows that the pulses having short rise/fall times have larger high-frequency content that do pulses with long rise/fall times.

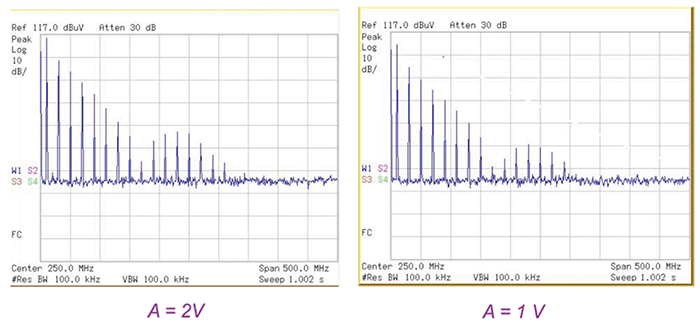
The effect of the signal amplitude on the frequency content of a trapezoidal signal is shown in Figure 6.
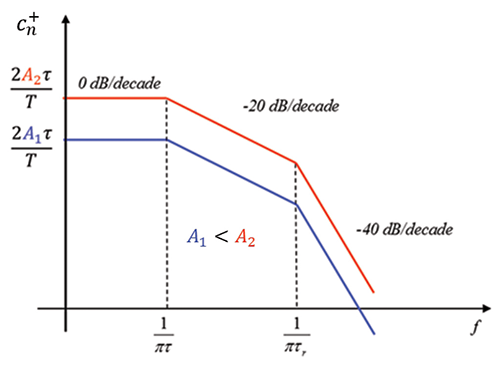
As can be seen, reducing the signal amplitude reduces the frequency content over the entire frequency range. This is verified by the measurement shown in Figure 7.
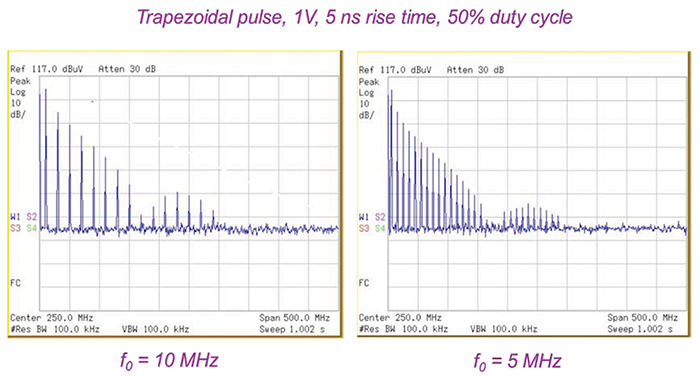
The effect of reducing the fundamental frequency while maintaining the same duty cycle on the frequency content of signal is shown in Figure 8.
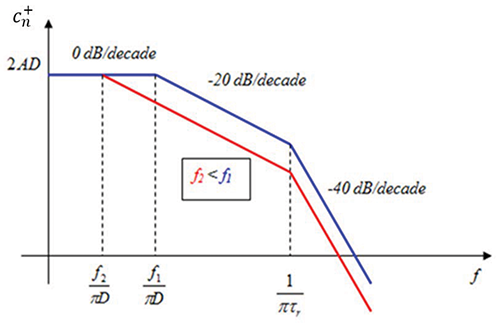
Reducing the fundamental frequency (while maintain the duty cycle) reduces the high-frequency spectral content of the waveform, but does not affect the low-frequency content. This is verified by the measurement shown in Figure 9.
Finally, the effect of reducing the duty cycle while maintaining the fundamental frequency is shown in Figure 10.
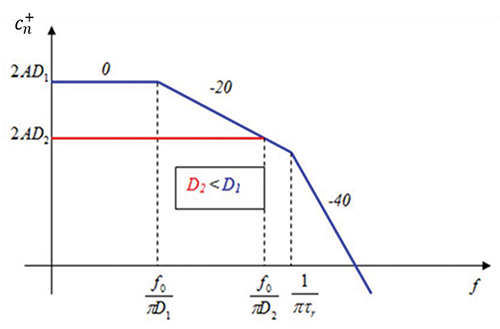
Reducing the duty cycle (the pulsewidth) reduces the low-frequency spectral content of the waveform, but does not affect the high-frequency content. This is verified by the measurement shown in Figure 11.
References
- Clayton R. Paul, Introduction to Electromagnetic Compatibility, Wiley, 2006.
- Bogdan Adamczyk, Foundations of Electromagnetic Compatibility with Practical Applications, Wiley, 2017.
