Understanding Radiated Emissions Measurements Made at One-Meter Separation: It’s Not What You’ve Been Led to Believe

Background¹

The first article in this series (see In Compliance Magazine, May 2023) described in detail the use of radios that used unshielded connections (termed antenna lead-ins) between the radio and the external antenna.² The antenna lead-in was a single wire above ground, using aircraft structure for a return path, that was connected to high impedances at both ends. That is, the radio input was the grid of a vacuum tube, so basically a small capacitance, and the external antenna was an electrically short wire over most of the 0.15 – 20 MHz (200 –15 meters) frequency (wavelength) range of the radio. 

Figure 1 shows a WWII-era handbook drawing of such an installation.³ Handbooks of the time went to great lengths showing photographs of poor and good radio antenna lead-in installations.⁴ Controlling the loop area of the lead-in over ground relative to that of adjacent culprit noise emitters (crosstalk) was emphasized.

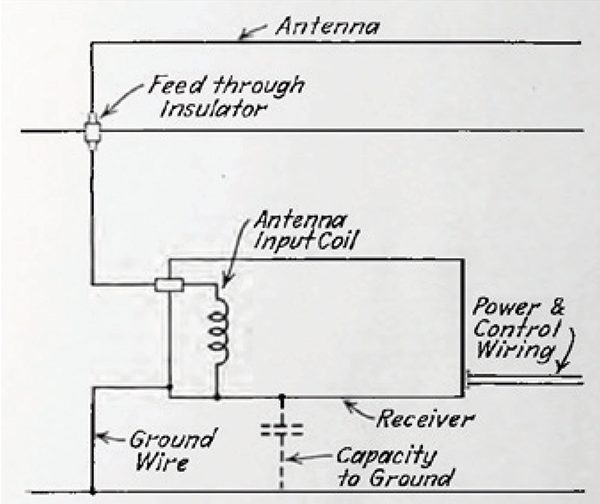
The specification that introduced EMI requirements based on controlling interference to/from these unshielded transmission lines was MIL‑I‑6181B, released in May 1953.⁵ As described in Reference 2, a report is available describing the selection of test antennas and set-ups for measuring to limits controlling radiated coupling to the unshielded antenna lead-in.⁶ NADC‑EL‑5515, released in 1955, is the rationale behind the radiated emission measurement limits and test methods of MIL‑I‑6181B. 

NADC‑EL‑5515 should be required reading for every vehicle EMC engineer. If the physics described in NADC‑EL‑5515 were universally understood by EMC engineers, there would be no need for the near field physics discussion in this article. Unfortunately, this knowledge is truly lost, and it is apparent from the state of aerospace (RTCA/DO-160 section 21) and automotive vehicle radiated emission EMI specifications (CISPR 25), and standards that support them (SAE ARP-958), that such understanding is sadly lacking.^7,8,9

Purpose

Hence, the true purpose of this article, which is only peripherally historical. The reader is requested to be patient, as much of what is presented will appear at first rather obvious. The more obvious, the better, because the conclusion is antithetical to most people’s thinking, and a surprising conclusion is much more convincing if the trail there is familiar and well-worn. The conclusion is this simple: only a far-field measurement results in a true field intensity measurement. That is, the signal level measured at the EMI receiver, adjusted for any losses/gains in the transmission line path, is only relatable to a specific field intensity when the antenna’s physical aperture is immersed in a field of constant amplitude across it. 

Under any other conditions (the near field) that relationship cannot be made, and any artificial attempts (one-meter field intensity limits supported by a one-meter antenna factor) are not only doomed to failure but also wrongheaded. This means that there is no valid use for such artificial constructs, and they lead to bad engineering decisions. This is not to say that near-field measurements are useless – far from it. A near-field measurement is absolutely necessary when the actual culprit-victim interaction is near field. But the point is, far- vs. near-field measurements are not simply quantitatively, but also qualitatively different.

We will start by defining and differentiating the concepts of far and near fields, all from an EMI test point of view. Far field is easiest, so we begin there.

Far Field 

The far field as an abstraction is a simple concept to visualize and understand intuitively. The near field is more complex, but as a starting point, we are in the near field when we are not in the far field. In terms of radiated emission measurements, the concept of far field is mostly associated with standards such as CISPR 22 and the newer 32.^10,11 These standards provide for test sample/antenna separations of 3 to 30 meters at and above 30 MHz. The far field assumption means limits scale directly with separation distance. It also means that – and this is key – these standards do not specify antenna types, only that they be calibrated in the far field. As we shall see, limit scaling with distance and the assumption that the same result may be obtained with any suitably calibrated antenna are hallmarks of a far-field measurement. Neither of these is true in the near field. And this is what MIL‑I‑6181B and NADC‑EL‑5515 first presented to the EMC world, and which remains true to the present day, albeit too few practicing EMC engineers appreciate it.

All of the following criteria need to be met in order to be in the far field of a transmitting source:

The far field is traveling electromagnetic energy. That means the far field propagates independently of the existence of the transmitting antenna once it is launched. The electric and magnetic field components of a traveling wave (Poynting’s theorem) are in contrast to the quasi-static and induction field components close to the antenna, which begin and end on antenna elements, and vanish when the antenna excitation is removed. An example of the independence of the traveling wave from its source is the light from a distant star reaching Earth. The star may in fact no longer exist, but the light it radiated away is still traveling through space. Closer to home, one necessary (but insufficient in and of itself) requirement for achieving the far field is being at or beyond the distance at which the amplitude of the traveling wave exceeds that of the quasi-static and induction components. Heinrich Hertz derived this criterion for an electrically short dipole.

A far-field traveling electromagnetic wave emanates from a point source. This means that the wave front has spherical curvature. This doesn’t mean that the radiating source is literally a point, which would yield not only spherical curvature but also spherical symmetry. It means the transmitting source is so far away from the observation point that it appears as a point; the distance from the observation point to any point on the transmit antenna is equal. This assumption is inherent in the derivation of the Hertzian short dipole field components. 

The wave front is not only spherical but also plane. Meaning that the sphere’s radius of curvature is large enough that over the physical aperture of our receive antenna there is no variation in field intensity or power density. The spherical wave front approximating a plane wave is the source of expressions for the far field such as 2D²/λ.

Now for the distance from an observation point to any point along an extended structure to be equal, the observation point must be infinitely far away. Similarly, a plane surface may be described as the surface of a sphere of infinite radius. But none of that is very practical for daily use. Instead, we decide on how closely our spherical surface needs to approximate a plane surface, typically by positing a maximum phase difference between any two lines from the observation point to different portions of the transmitting source. Figure 2 shows this process specifying the allowable variation from a plane wave as a fraction of a wavelength.

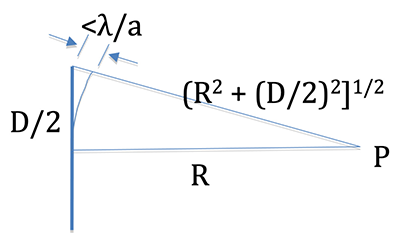
In Figure 2, the circular arc struck between the radius labeled R and the radius that is its hypotenuse marks off the length of the hypotenuse that is greater than R and denotes it as a fraction of a wavelength. The value we assign to “a” depends on the application of interest.

Setting

R + λ/a = √(R² + (D/2)²)

and solving for R, we get

R = (a/2λ) (D/2)² – λ/2a  or 

R = aD²/8λ – λ/2a

When we pick a maximum phase front variation of a sixteenth wavelength (a = 16), we get 

R = 2D²/λ – λ/32

In order for this to approximate the familiar 2D²/λ, the first term must greatly exceed the second term. This is certainly the case when the transmit antenna is at least a half‑wavelength long. 

From this analysis, in addition to learning/reviewing the derivation for 2D²/λ, we understand that it is based on a traveling electromagnetic wave far from the point source. We can also see that if the antenna is electrically short, the derivation doesn’t work at all. The phase difference between the two radial distances in Figure 2 will always be smaller than the phase difference associated with distance d/2. If the antenna dimensions are an insignificant fraction of a wavelength this problem formulation says the far field is at any distance from the antenna, including zero. That is, if the phase difference from the center of the antenna to its end is the same or smaller than the phase difference we posit as acceptable for the far field criterion, then even a point on the antenna centerline is in the far field in terms of the above analysis. This merely emphasizes that the analysis assumes an electrically long antenna and a traveling electromagnetic wave.

Near Field

Now let’s look at the opposite situation: the one-meter radiated emission measurements that are very similar between MIL‑STD-461, and RTCA/DO‑160 section 21 and CISPR 25.¹² In these standards, minimum lengths of cables vary from 1.5 m (CISPR 25) to 3.3 meters (RTCA/DO‑160). This means that the antenna separation from the radiating structure is much less than the length of the radiating structure. 

Figure 3a demonstrates that the radiating structure is not a point source.  Further, the dimensions of the antennas used below 1 GHz are on the order of the separation distance, so that the test antenna is not measuring a single, constant amplitude of field intensity over its physical aperture, but instead is integrating a complex variation of field intensity over its physical length. Figure 3b shows the radiation situation most people visualize when making antenna measurements. Figure 3c is similar to Figure 3a and in direct contrast to Figure 3b, showing the extreme near field. Figure 3c is an end view of the isometric view shown in Figure 3a, showing the electric field due to a wire over a ground plane. 

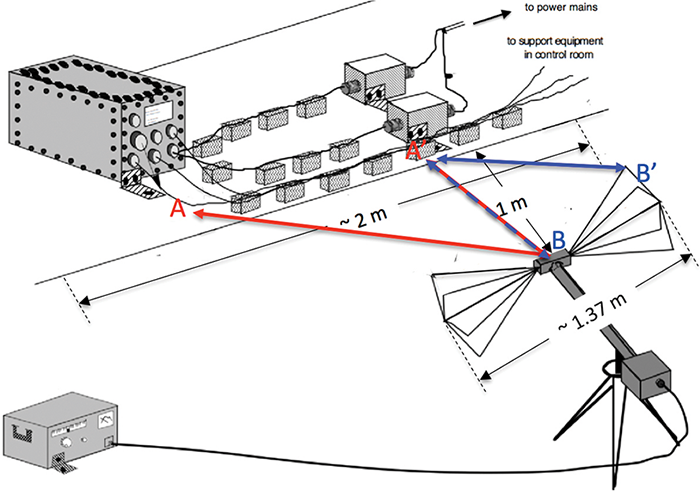
Figure 3c emphasizes that antenna placement is critical. Placement of identical antenna types at various positions from A to C reveals that the received signal will be dependent on the orientation of field lines, which is strongly a function of the position close to the test sample. Inspection of two of the same type antennas with different lengths at position D emphasizes that the longer antenna is not measuring constant field intensity over its physical aperture, but instead is integrating a variation of field intensity over its physical length. We cannot predict from the position D measurement with the smaller antenna what the larger antenna would measure because while the smaller antenna is illuminated by a near-constant amplitude electric field, the larger antenna is not. And we cannot extrapolate from the measurement with the larger antenna to using the same antenna or another antenna at another position, for the same reason. 

In Figure 3b, the voltage measured at the antenna port where the phase front is constant over the antenna physical length is directly proportional to the field intensity impinging upon it. In contrast, in Figure 3c the single value of “field strength” derived from the voltage at the antenna is only representative of what is measured at this particular position relative to the test sample, and using a particular antenna, at a particular orientation. The measured value is not a scalable far field “field intensity” in the sense that one can use it to predict the field intensity at some other distance or measured with a different antenna. 

A real-world example of these limitations is FCC rules for EMI testing on an open area test site (OATS) back in the 1980s. The original test method used half-wave dipoles at a three-meter separation from the test sample. At 30 MHz, this placed a 5-meter-long antenna at 3 meters distance. The antenna was longer than the separation: near field. When biconicals started to become popular as tunable dipole replacements, the FCC ruled that while biconicals were acceptable, if there were any question about whether the test sample met the limit, a dipole measurement would be made, and that result would be used. The ~140 cm biconical was actually a better far-field measurement due to its much shorter length, but the point was that a standard had to be maintained, and they knew that biconical and 30 MHz half-wave dipole measurements would not agree.

A final note about Figures 3a, 3b, and 3c: The issues illustrated are frequency independent. Regardless of frequency – dc to light – if the above conditions apply, the measurement is near field. 

In the words of NADC‑EL‑5515, referring to antenna types used for EMI testing in the 1950s:

“For instance, a resonant dipole antenna has good sensitivity to low-impedance (magnetic) fields near its center and high-impedance (electric) fields near its ends. Other antennas, such as the discone, have a completely different distribution of impedance, polarization, physical size, and contour. Obviously, a comparison of the effect of a given interference field upon the dipole and the same field on a discone can only be made in a very general manner. For this reason, (MIL‑I‑6181B) radio interference limits are derived expressly for each particular antenna that is to be used, and an exact correlation between different types of antennas is not expected.”¹³ 

This is the end of the first installment of
“(Re)Discovering the Lost Science of Near Field Measurements.” Future installments will include sections on:

• Describe, compare, and contrast near field antenna-induced vs. field intensity limits
• Introduction and detailed description of the meaning of antenna-induced signals
• Rationale behind the switch from antenna-induced to field intensity EMI limits
• Technical problems incurred using field intensity limits in the extreme near field
• Practical problems resulting from using field intensity limits where antenna-induced is more appropriate 

Endnotes

1. All standards and specifications referenced herein which are not copyrighted are available from http://www.emccompliance.com.
2. Javor, Ken, “Seventy Years of Electromagnetic Interference Control in Planes, Trains, and Automobiles,” In Compliance Magazine, May 2023.
3. Also see Figures 2 and 3 of Reference 2.
4. NAVAER 16-5Q-517, Elimination of Radio Interference Problems in Aircraft, circa 1946-47.
5. MIL‑I‑6181B, Interference Limits, Tests and Design Requirements, Aircraft Electrical and Electronic Equipment, 29 May 1953.
6. NADC‑EL‑5515, Final Report, Evaluation of Radio Interference Pick-Up Devices and Explanation of the Methods and Limits of Specification No. MIL‑I‑6181B, 10 August 1955.
7. RTCA/DO-160, original through C revision, Environmental Conditions and Test Procedures for Airborne Equipment.
8. CISPR 25, all editions, various titles. “Limits and methods of measurement of radio disturbance characteristics for the protection of receivers used on board vehicles” is the 1995 title.
9. SAE ARP-958, all revisions, Broadband Electromagnetic Interference Measurement Antennas; Standard Calibration Requirements and Methods, 01 March 1968.
10. CISPR 22 – Information technology equipment – Radio disturbance characteristics – Limits and methods of measurement (withdrawn).
11. CISPR 32 – Electromagnetic Compatibility of multimedia equipment – Emission requirements.
12. MIL-STD-461E, and newer, Requirements for the Control of Electromagnetic Interference Characteristics of Subsystems and Equipment, 1999-present.
13. A discone antenna is half of a biconical, mounted vertically above or below a ground plane that provides the missing biconical element as an image in the ground plane.