Effect of Multi-Burst Lightning Flashes on Surge Protective Devices Using MOVs

The story of the multi-burst flash begins with Karl Berger, a professor of high voltage engineering at ETH Zurich. He was known as the “father of lightning research” for his pioneering observations at the lightning-detection station on Monte San Salvatore from 1943 to 1972. His work is important because early on it showed that a lightning flash consisted of a burst of a first stroke followed by several subsequent strokes, all spaced a few milliseconds apart, as illustrated for example in his 1967 paper [1].

As a result of Karl’s work, the structure of a lightning flash is now well-known, which would suggest that multi-burst lightning testing should now be routinely done. But only a few labs have constructed equipment to do multi-burst testing (e.g., Darveniza and co-workers in Australia, Ray Hill and co-workers at Georgia Tech, and recently Zhang and his co-workers in China). So why hasn’t multi-burst testing to simulate lightning been more widely done? And why hasn’t it made its way into standards?

Why Conduct Single Large Surge Testing?

One possible reason goes back to the observation by Bodle et al. in 1976 [2] that,

“For design tests of the lightning withstand capability of plant items and associated equipment, both in the communication and power industries, a single large impulse is employed. This is an ‘equivalency’ type of test dictated by practical test considerations. Experience has indicated, however, that this is an acceptable simulation of actual field exposure, which includes multiple component strokes.”

So what Bodle and his co-authors are saying is that, yes, we know about multiple component flashes, but multiple-surge testing isn’t needed because single large-burst testing works well enough. That would probably be true if they were testing gas tubes or carbon blocks, which were the main types of surge protection devices (SPDs) used in 1976.

A second possible reason is that equipment to conduct multi-burst testing is not commercially available, and would likely be expensive if it were. Either way, multi-burst testing is generally not done on SPDs.

What We Miss, and Why Multi-Surge Burst Testing Needs to be Done

So what do we miss by testing with a single large surge, or multiple but widely-spaced surges spaced (which looks like a series of single surge tests)? If we’re testing switching SPDs (e.g., gas tubes or thyristors), then there may not be a problem. But there could be with clamping SPDs because clamping SPDs, especially metal oxide varistors (MOVs), can have a long thermal time constant. Long thermal time constants can cause heat accumulation in an SPD subjected to a multi-surge burst (possibly including continuing current). Heat accumulation can lead to a potentially destructive temperature rise, which is what we miss when a multi-surge burst test is replaced by a single large surge, or multiple surges spaced widely apart.

Figure 1 shows an example of a lightning flash where we might expect heat accumulation to occur.

The view that multi-surge burst testing is needed is supported in a paper by Sargent et al. [4]. In their study, half a set of 18 mm MOV samples were subjected to a multi-surge burst of 8/20 surges at rated current. These samples showed signs of damage, whereas the other half of the samples tested with a single 8/20 surge at rated current repeated at intervals of 60 seconds or more showed no damage. In another multi-surge burst test, Rousseau et al. [5] subjected a MOV to sixty 20 kA 8/20 surges spaced 60 seconds apart, with no failure. But when the same type of MOV was subjected to as few as five 20 kA 8/20 surges spaced 50 ms apart, failure occurred.

For the most part, the multi-burst flash work on clamping SPDs has been done on MOVs, because MOVs have a long thermal time constant. Due to the long time constant, the energy deposited in a MOV from one of a series of closely-spaced surges might not dissipate before the next surge arrives, allowing energy to build up. The same is true for silicon-based devices but to a lesser extent because the thermal time constants for silicon devices are shorter than for MOVs. So we’ll concentrate on MOVs; and for an illustrative example, the application of MOVs to the protection of DC feeds to remote radio heads (as discussed in [6]).

Thermal Modeling of MOVs

Since we’re interested in heat accumulation, we need to look into thermal modelling. Due to the non-uniformity of the material of an MOV, current tends to concentrate in a relatively small conduction channel in the MOV. In the study of Sargent et al, analysis of the failed MOV samples showed cracking and a formation of new amorphous material near the conduction channel. Examination of this amorphous material suggested that local hot spots (actually, hot channels) formed when a current pulse was passed through the MOV. The amorphous material in these hot spots likely resulted from a plasma formed during the current pulse, which rapidly cooled afterward due to heat conduction to the surrounding ZnO grains.

From X-ray fluorescence analysis of the amorphous material, creation of the amorphous material was thought to require a local temperature around 1000^o C. Thermal modelling suggested that this temperature rise would occur if the pulse power was concentrated in about 2 percent of the MOV volume. This is an important observation because a calculation of the energy absorbed in the multi-surge burst test showed that the temperature rise of the MOV would only have been 231^o C if the temperature distribution were uniform, much less than the temperature thought to have caused the damage.

The results of Sargent et al suggest that the criterion for failure of an MOV is a localized temperature rise to 1000^o C (or the vicinity thereof). So, for an MOV under consideration, we need to determine if a localized area might reach 1000^o C. To do that we begin with a thermal model, as shown in Figure 2.

Thermal paths can be modelled using an electrical circuit analog. In thermal modelling, Temperature T(t) in ^o K corresponds to volts in an electrical circuit, and Power P in watts corresponds to current. Keep this in mind as we look at the electrical circuit analog of an MOV shown in Figure 2.

In Figure 2, R₁ and C₁ are the thermal resistance and thermal capacitance of the area that might reach 1000^o C, and R₂ and C₂ are the thermal resistance and thermal capacitance of the rest of the MOV.

From the electrical circuit analogy, we can write the thermal impedance Z(ω) as

  (1)

where

(R₁ and R₂ being the thermal resistances)

and

s=jω

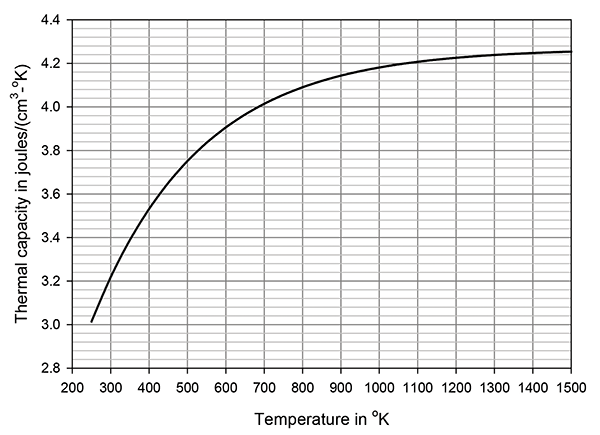
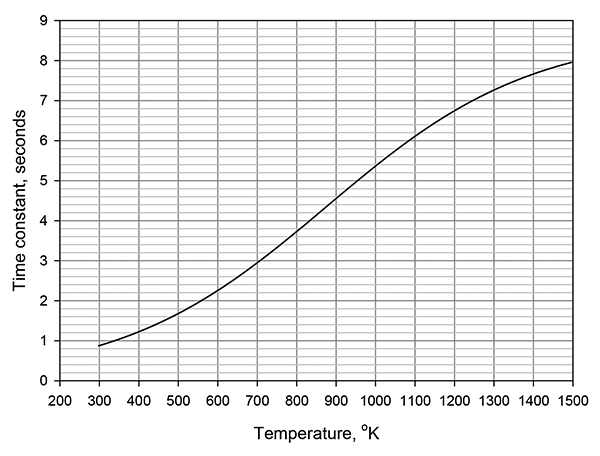
For zinc oxide (the basic component of MOVs), both the thermal conductance and the thermal capacity are functions of temperature, as shown in Figure 3 and Figure 4 (both based on data in Wiley books [7]). Due to that temperature dependence, the thermal time constant is also a function of temperature, as shown in Figure 5.

Computing the Temperature Rise of an MOV for Single Surges

Referring to Figure 2, assume the power W(t) is given by:

   (2)

Where I_p is the peak current of the surge, and V_mov(i) is the MOV voltage as a function of current.

In the frequency domain equation (2) is

   (3)

The temperature rise T(ω) is then

    (4)

In the time domain, T(t) is

    (5)

where

Example Calculation – the Temperature Rise of an MOV for a Single Surge

As an example of a temperature-rise calculation, consider the protection of dc feeds to remote radio heads (RRH), a prominent application for MOV SPDs discussed in [6]. Suppose we have a tower 80 m tall having a RRH 48 V DC feed we want to protect against lightning. For lightning, assume a flash which has a downward leader and negative polarity, since about 90 percent of all lightning is of this type. It characterizes ground flashes and flashes to towers generally less than 80 m tall. A typical flash of this type is composed of three to five strokes, with typical interstroke intervals of 30 – 60 milliseconds. It might also contain continuing current and, in some cases, initial current.

Let’s be conservative and assume a lightning flash with five percent probability of occurring. Then from CIGRE TB549 [8], the amplitude of a first stroke is 80 kA and the action integral (i²t) is 5.5×10⁵ A²s. If we assume a double-exponential waveshape, the corresponding time to half peak is calculated to be 120 µs. The rise-time is generally not correlated with the duration, and from [8] could reasonably be anything from 5.5 to 18 µs. Assume it is 10 µs (usually results are not sensitive to this choice). The waveform we have is then an 80 kA 10/120. Using the calculations from [6], an 80 kA 10/120 strike to an 80 m tower will result in a 17.5 kA 10/63 surge on the DC feed.

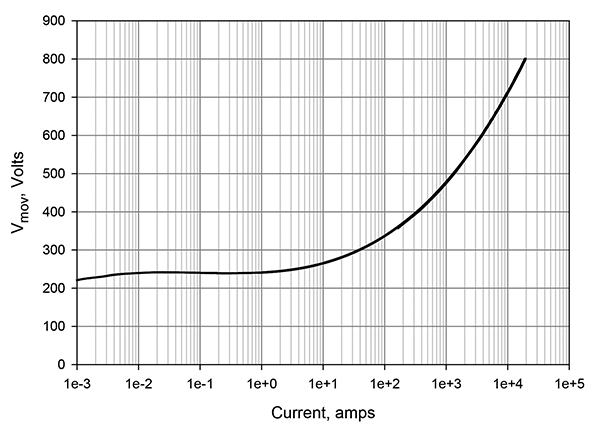
It appears that a 25 mm MOV rated at 20 kA with a Mcov of 130 V would be appropriate for the application, so we’ll select that. For the calculation, we need the V-I curve for this device. This curve depends on the device manufacturer, but the higher current region for a typical MOV of the type chosen is shown in Figure 6.

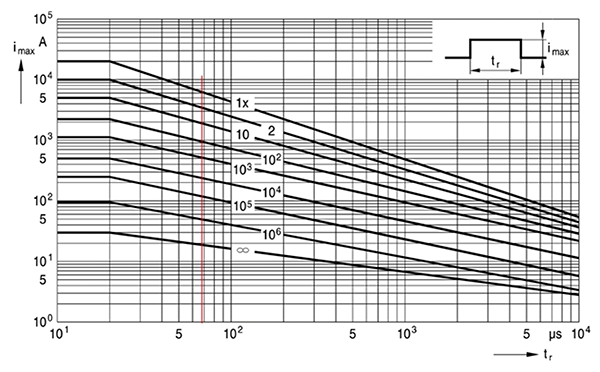
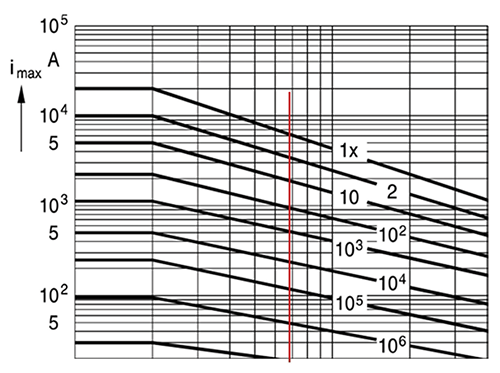
One other piece of information we need is the current vs. surge-width derating curves for the chosen MOV. In the present case, a typical set is shown in Figure 7, and an expanded view in Figure 8.

Ok, we’ve calculated the wire current as 17.5 kA, and the chosen MOV is rated at 20 kA, so we’re good to go, right?

Well, no! The 20 kA rating of the chosen MOV is for an 8/20 surge, and ours is 10/63. To find the rating for a 10/63 surge, we need to consult the derating curves in Figure 7. To use these curves, we need to convert the current-time relation of a double-exponential to that of a rectangular pulse of width t_r. The conversion factor is t_r = (a – b)/ab, where a and b are as shown in equation (2). For a 10/63 surge, t_r = 69 µs. In Figure 7 (or Figure 8), if we follow up the red line at t_r = 69 µs to where it intersects the curve for a single surge, I_p = I_max = 6 kA, which is substantially less than the 17.5 kA expected from an 80 kA strike. So, if we want to handle the 17.5 kA surge on the dc feed we would need to put at least three of these devices in parallel so that no single device has to handle more than 6 kA.

Assume enough of the devices are in parallel such that no single device handles more than 6kA. For the device considered, the conductivity and heat capacity can be read from Figure 3 and Figure 4. Figure 6 is used to calculate Vmov(i) as a function of current. Remembering that the power surge is of the form e^-at – e^-bt, for a 10/63 surge, a = 1.39×10⁴ and b = 3.12×10⁵. Then from equation (5) we can then calculate the temperature rise in one MOV for a 6 kA 10/63 surge, and plot the result (see Figure 9).

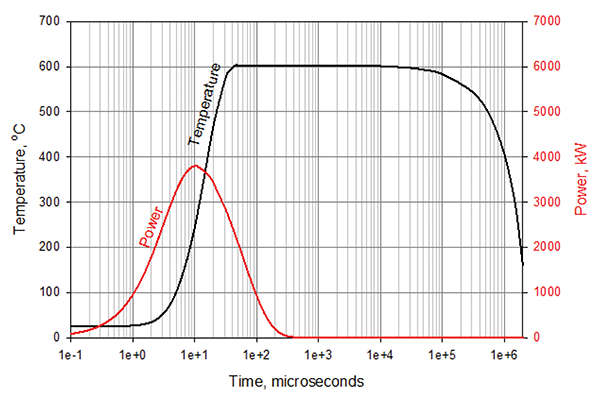
Keeping in mind that the finding of Sargent et al that MOV failure occurs when the temperature in the channel area reaches the vicinity of 1000^o C, Figure 9 indicates that failure is not expected for the single-shot case considered. So, what about a multi-burst flash?

Example Calculation – the Temperature Rise of an MOV for Multiple Surges

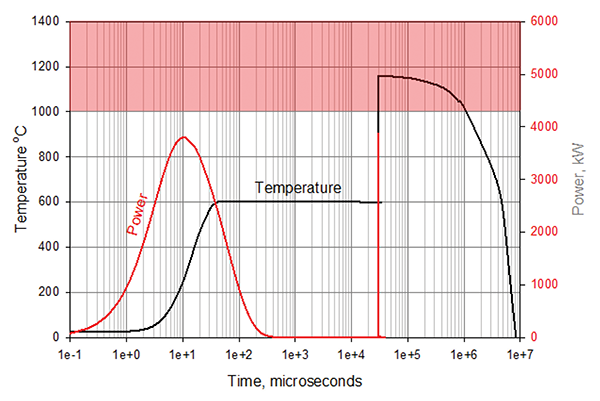
Figure 10 shows the additional temperature rise that happens when the surge used to create Figure 9 is applied to the MOV a second time after 30 ms (the surge at 30 ms is identical to the first one, but shows up as a line due to the logarithmic time scale). The additional temperature rise is due to the relatively long thermal time constant of the MOV, which prevents the MOV from dissipating much heat energy before the second surge arrives. The temperature rise is now in the red area above 1000^o C, where failure is expected. This result is consistent with the derating curves of Figure 8, which indicates that more than one surge at 6000 A would lead to failure.

Figure 8 suggests that no failure would occur for two surges of 10/63 if I_max were reduced to 3400 A which, all else being equal, would require doubling the number of paralleled devices. Calculations suggest that two surges of 3400 A 10/63 spaced 30 ms apart would result in a temperature rise of 630^o C, well below the assumed 1000^o C failure level. This is just an example, and what happens in an actual application depends on the relevant surge amplitude and the number of the surges.

In the example calculation, the total number of surges was two, but in an actual application the total number of surges will be many more. The number of surges can be estimated by assuming n number of lightning flashes per year (from an isokeraunic map), having a number of strokes per flash x, and setting the number of years of expected service at y, for a total of nxy surges. So, for example, if the service life of an RRH equipment is 20 years, and 10 lightning flashes containing five strokes occur per year, then the total number of surges is 1000. From Figure 8 characterizing the MOV chosen, running up the red line for 1000 10/63 surges, I_max would be limited to about 500 A, so a much larger MOV should be chosen.

Comments

There are many variables in this analysis, and conclusions could change depending on the assumptions used. In particular, we assumed that all surges have the same amplitude (to make it easy to use the derating curves). Generally, there would be subsequent surges of lower amplitude and duration, but assuming all surges have the same amplitude and duration as the first one is a worst-case, unless there is continuing current or initial continuing current

In the general case, the procedure used to calculate temperature rise can still be used, if the exact sequence and characteristics of the first surge, subsequent surges, and any continuing current or initial continuing current is known. The power input from each event in the flash is added to the previous one (with the appropriate time delay), and the cumulative temperature rise calculated. If the calculated temperature rise exceeds 1000^o C (or more conservatively, 800^o C), then failure can be expected.

This calculation for an arbitrary sequence of surges and continuing current involves a lot of work, and is probably not worthwhile to do except for forensics. Forensic studies have been done, for example, that of Yang et al. [9] with comments in [10], where the issue was why a 40 kA rated MOV failed when subjected to a series of surges, none of which exceeded 26 kA, and continuing current.

Practical Procedure for Selecting an MOV

No Continuing Current Present

If there is no continuing current (for example the case of DC feeds to RRH considered above), the following procedure could be helpful for selecting an MOV:

• Consult an isokeraunic map of your area to estimate the number of lightning flashes, n, expected in a year;
• Estimate the service life, y years, of the equipment to be protected; then
• Estimate the number of strokes, x, in the flash. If unknown, four or five is a good guess.

With that information, the total number T_L of surges expected is n times x times y.

• Determine the waveform of the surge to be protected against:
  • If a standard applies, use that;
  • If the equipment is an RRH, reference [6] might be helpful;
  • CIGRE TB549 is a useful reference;
  • If none of the above works, a possible fallback is to assume an 80 kA 10/120 low probability stroke for extreme environments (like towers), or a 30 kA 5.5/75 median probability stroke for less exposed environments.
• Assuming your surge is a double-exponential like that in equation 2, calculate t_r = (a – b)/ab;
• Choose an MOV. On the derating curve for the MOV, go to t_r and move up to the curve for number of surges = T_L. Read across to the maximum allowed current Imax;
• If Imax is greater than the peak current for the waveform you have assumed or calculated, you’re good to go. If Imax is less than the peak current for the waveform you have chosen, you will either need to parallel enough devices to handle the current, or choose a bigger MOV.

Continuing Current Present

Sometimes the continuing current is enough by itself to cause failure. To see if that is the case, approximate the continuing current by a rectangular pulse having the amplitude Imax and a duration t_r of the continuing current. On the derating curve for the MOV, look for the intersection of Imax and t_r (the derating curve may need to be extended to find this). If it is above the curve for a single surge, then failure will occur.

Conclusion

MOVs are the SPDs most susceptible to failure due to multi-burst surges. In the case of MOVs, inhomogeneity in composition causes current surges to be conducted in a narrow channel. The confined surge causes a temperature rise which if it exceeds 1000^o C can cause failure. Heat accumulation due to multi-burst surges may cause a temperature rise in MOVs to exceed 1000^o C, leading to failure. That suggests that at least for critical applications, multi-burst surge testing should be done.

Endnotes

The analysis here applies to any sequence of closely-spaced surges, not just lightning. In addition to MOVs there are also silicon clamping devices. They too can suffer from accumulated temperature effects. But silicon devices have a much different thermal time constant, a different thermal model, and a different failure mode, so that’s another story.

References

1. 1. Berger, K., “Novel Observations on Lightning Discharges: Results of research on Mount San Salvatore,” J. Franklin Institute, vol. 283, pp. 478-525, 1967.
   2. Bodle, D. W., A. J. Ghazi, M. Syed, and R. L. Woodside, Characterization of the Electrical Environment, Toronto: University of Toronto Press, 1976.
   3. Rakov, V. A., “Lightning Parameters of Engineering Interest: Application of Lightning Detection Technologies,” in EGAT, Bangkok, Thailand, November 7, 2012.
   4. Sargent, R. A., G. L. Dunlop and M. Darveniza, “Effects of Multiple Impulse Currents on the Microstructure and Electrical Properties of Metal-oxide Varistors,” IEEE Transactions on Electrical Insulation, vol. 27, no. 3, p. 586, 1992.
   5. Rousseau, A., Zhang, X., and M. Tao, “Multiple shots on SPDs – additional tests,” in Int’l Conf. on Lightning Protection (ICLP), Shanghai, 2014.
   6. Martin, A. R., “Power feeds to remote radio heads – what protectors need to deal with,” in The Alliance for Telecommunications Industry Solutions Protecteion Engineers Group Conference, Monroe, LA, 2016.
   7. www.wiley-vch.de/books/sample/3527408134_c01.pdf
   8. CIGRE WG C4.407, “TB549 Lightning Parameters for Engineering applications,” 2013.
   9. Yang, S. J., S. D Chen, Y. J. Zhang, W.S. Dong, J. G. Wang, M. Zhou, D. Zheng, and H. Y Hui , “Triggered Lightning Analysis Gives New Insight into Over Current Effects on Surge Protective Devices,” www.ten350.com/papers/icae-conghua.pdf.
   10. M. Maytum, “CIGRÉ (Council on Large Electric Systems) Technical Bulletin (TB) 549 (2013) Lightning Parameters for Engineering Applications,” in The Alliance for Telecommunications Industry Solutions Protecteion Engineers Group Conference, Littleton, CO, 2014.