This article presents a concept of a wave together with the wave equations and their solutions. The time-varying EM fields and their propagation in both the time and frequency domains is discussed first. Subsequently, the equations for the voltages and currents on the transmission line are obtained. It is shown that these equations and that their solutions represent voltage and current waves propagating along the line.
1. Concept of a Wave
Consider a function of time t and space z, , with its argument given by
![]() (1.1)
(1.1)
Then,
![]() (1.2)
(1.2)
Eq. (1.2) is valid for any ∆z and any ∆t. Thus, we could choose any relationship between the two deltas, and the new equation would still be valid. Let’s choose this relationship to be
![]() (1.3)
(1.3)
Then, Eq. (1.2) becomes
![]() (1.4a)
(1.4a)
or
![]() (1.4b)
(1.4b)
Therefore, after a time ∆t, the function f retains the same value at a point that is ∆z = v∆t away from the previous position in space (defined by z), as shown in Figure 1.
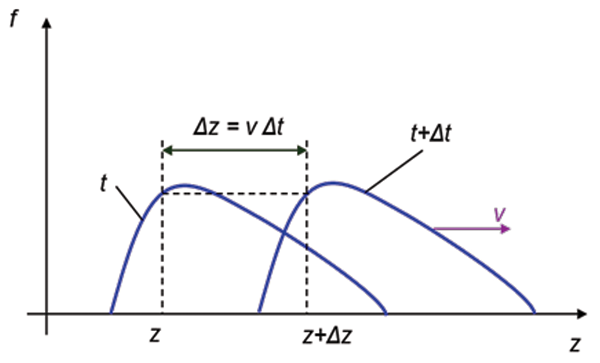
This means that any function of the form represents a wave traveling in the positive z direction with a velocity
![]() (1.5)
(1.5)
Similarly, it can be shown that any function of the form f (t ‒ z/v) represents a wave traveling in the negative z direction as the time advances.
2. Uniform Plane EM Wave in Time Domain
The time variations of the magnetic (H) and electric (E) fields give rise to the space variations of the electric and magnetic fields, respectively. This interdependence of the space and time variations gives rise to the electromagnetic wave propagation.
The two fields are related by Maxwell’s equations (in source-free medium)
![]() (2.1a)
(2.1a)
![]() (2.1b)
(2.1b)
where µ, σ, and ε are the permeability, conductivity, and permeability of a medium, respectively.
In general, the electric and magnetic fields have three nonzero components, each of them being a function of all three coordinates and time. That is,
![]() (2.2a)
(2.2a)
![]() (2.2b)
(2.2b)
We will focus on a simple and very useful type of wave: uniform plane wave. Uniform plane waves not only serve as a building block in the study of electromagnetic waves but also support the study of wave propagation on transmission lines as we will show.
Under the uniformity in the plane assumption, if the E field points in the +x direction (usual designation) then the Maxwell’s equations show that the H field is pointing in the + y direction, and
![]() (2.3a)
(2.3a)
![]() (2.3b)
(2.3b)
This is shown in Figure 2.
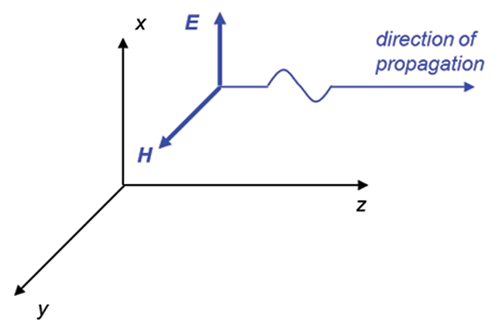
The fields propagate as waves in the positive + z direction. Under the uniformity in the plane assumption, Equations (2.1) for a lossless medium (σ = 0) become
![]() (2.4a)
(2.4a)
![]() (2.4b)
(2.4b)
and their general solution, in a lossless medium, is [1],
![]() (2.5a)
(2.5a)
![]() (2.5b)
(2.5b)
where
![]() (2.6)
(2.6)
is the intrinsic impedance of a (lossless) medium, and A and B are constants.
Based on the discussion in Section 1, we recognize the functions f and g, as waves propagating in +z and -z directions, respectively, with a velocity of propagation equal to
![]() (2.7)
(2.7)
3. Uniform Plane EM Wave in Frequency Domain
In the previous section, we described the wave equations in a lossless medium for arbitrary time variations. When the time variations are sinusoidal, the wave equations in any (simple) medium become [1]:
![]() (3.1a)
(3.1a)
![]() (3.1b)
(3.1b)
where
![]() (3.2)
(3.2)
is the propagation constant of the medium. The general solution of Equations (3.1) is
![]() (3.3a)
(3.3a)
![]() (3.3b)
(3.3b)
where
![]() (3.4)
(3.4)
is the intrinsic impedance of the medium. The propagation constant is often expressed in terms of its real and imaginary parts as
![]() (3.5)
(3.5)
where α is the attenuation constant and β = w/v is the phase constant. The complex intrinsic impedance is often expressed in an exponential form as
![]() (3.6)
(3.6)
Then the solution in Equations (3.3) can be written as
![]() (3.7a)
(3.7a)
![]() (3.7b)
(3.7b)
Often, the undetermined complex constants can be expressed as
![]() (3.8a)
(3.8a)
![]() (3.8b)
(3.8b)
Then, the solutions in Equations (3.7) become
![]() (3.9a)
(3.9a)
![]() (3.9b)
(3.9b)
The corresponding time-domain solutions, in a lossless medium, are [1]:
![]() (3.10a)
(3.10a)
![]() (3.10b)
(3.10b)
Note that
![]() (3.11a)
(3.11a)
![]() (3.11b)
(3.11b)
Thus, the Equations (3.10) represents sinusoidal traveling wave in the +z, and -z direction, respectively!
Figure 3 shows a forward propagating EM wave in a lossless medium.
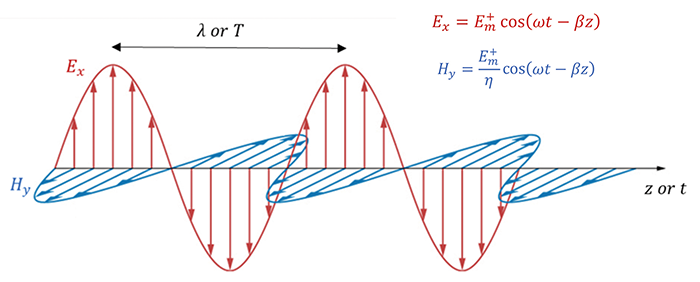
The wavelength λ is related to the velocity of propagation and frequency by
![]() (3.12)
(3.12)
The phase constant β is related to λ by
![]() (3.13)
(3.13)
From Eq. (3.13) we obtain
![]() (3.14)
(3.14)
We refer to z as a physical length and to z/λ as the electrical length. The time domain solutions in Equations (3.10) can now be written in terms of the electrical length as
![]() (3.15a)
(3.15a)
![]() (3.15b)
(3.15b)
The definition of electrical length leads to the concept of the electrically short structures.
Physical object is electrically short if its electrical length z/l ≤ 1/10 or equivalently if its physical length z ≤ l /10.
If the physical object is electrically short, then the lumped-parameter circuit models are an adequate representation of that object. This also means that we can use Kirchhoff’s laws instead of Maxwell’s equations to analyze the circuit models.
4. Voltage and Current Waves along a Transmission Line
In this section, we show that the voltages and current signals propagate as waves along a transmission line.
To obtain the transmission line equations, let’s consider a single segment of a lossless transmission line shown in Figure 4.
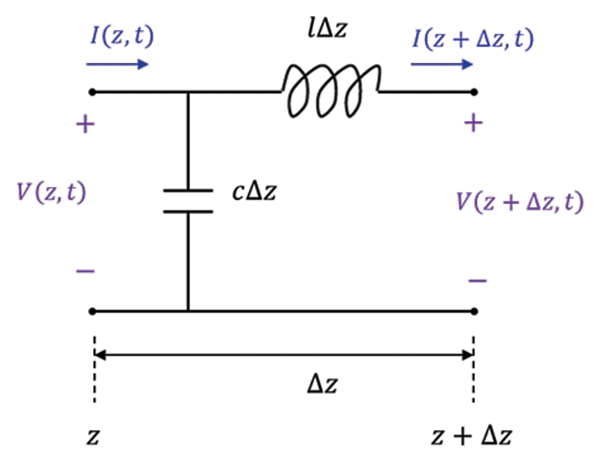
The distributed parameters describing the transmission line are: l – inductance per-unit-length (H/m) and c – capacitance per-unit-length (F/m).
Writing Kirchhoff’s voltage law around the outside loop results in
![]() (4.1)
(4.1)
or
![]() (4.2)
(4.2)
Dividing both sides by ∆z and taking the limit gives
![]() (4.3)
(4.3)
or
![]() (4.4)
(4.4)
Writing Kirchhoff’s current law at the upper node of the capacitor results in
![]() (4.5)
(4.5)
or
![]() (4.6)
(4.6)
Dividing both sides by ∆z and taking the limit gives
![]() (4.7)
(4.7)
or
![]() (4.8)
(4.8)
Equations (4.4) and (4.8) constitute a set of first-order coupled transmission line equations. These equations can be decoupled and expressed as [1]:
![]() (4.9a)
(4.9a)
![]() (4.9b)
(4.9b)
Compare the Equations (4.9) to the Equations (2.5) describing the EM wave. These two sets of equations have the same mathematical form. This means that the solutions of these two sets will have the same mathematical form! This also means that the voltage and current propagate as waves along the transmission lines!
The general solutions to the transmission-line equations (4.9)
are [1]:
![]() (4.10a)
(4.10a)
![]() (4.10b)
(4.10b)
ZC is the characteristic impedance of the line
![]() (4.11)
(4.11)
The function V +(t ‒ z/v) represents a forward-traveling voltage wave traveling in the +z direction, while the function V ‒(t + z/v) represents a backward-traveling voltage wave traveling in the -z direction.
Similar statements are valid for the current waves. The total solution consists of the sum of forward-traveling and backward-traveling waves.
The velocity of the wave propagation along the line is given by
![]() (4.12)
(4.12)
References
- Bogdan Adamczyk, Foundations of Electromagnetic Compatibility with Practical Applications, Wiley, 2017.
