A few months ago, we built a clear plastic box containing a four-inch square steel plane, a two-inch diameter steel sphere, and a micrometer drive to precisely adjust the distance between the plane and the sphere.
Recently, we finally had the time to do some testing. We set the distance to a known value and then slowly applied the voltage from a hi-pot tester until the tester tripped. We repeated the process for increasing distances. At each distance, we repeated the test at least once to determine consistency. The repeatability was about 50 volts rms or dc. (Subsequently we learned that the repeatability was related to the resolution of the hipot voltage control itself.)
We performed the test with both dc and 60 Hertz sinusoid waveforms.
Table 1 gives our test data. For ac, we measured the rms value and then calculated the peak value as 1.414 times the rms value. (We confirmed that the ac waveform was sinusoidal by observing the waveform with an oscilloscope.) The table includes ac (50 Hz) and 1.2 x 50 impulse breakdown voltages data from IEC 664.

Table 1
Plotting these data, we find that the ac peak and dc lines virtually overlay each other. (Figure 1)

Figure 1
Conclusion: In air, there is no difference in breakdown voltage between ac peak and dc voltages (for mains ac frequencies). This conclusion is incontrovertible. Note that this experiment is a 60 Hertz test, while IEC Publication 664, Table AII, is a 50 Hertz test. The 60 Hertz peak breakdown voltage is in good agreement with the 50 Hertz peak breakdown voltage up to about 5 kilovolts. Further note in IEC 664, Table AII, that the 50 Hertz peak breakdown voltage closely agrees with the 1.2 x 50 impulse breakdown voltage.
When we add these data to the graph, we find that there is still good agreement (although not as good as the ac to dc agreement) between the measured breakdown data and the lEC breakdown data. (We’ll examine the degree of disagreement more thoroughly a bit later.)
Conclusion: In air, there is no difference in breakdown voltage between dc, peak ac (either 50 or 60 Hertz), and 1.2 x 50 µsec impulse voltages.
Hypothesis: The breakdown of air is an absolute function of voltage and is not related to the waveform.
Conclusion: The breakdown of air is a linear function of the distance between the two electrodes.
Conclusion: For voltages below some value, air will not break down regardless of distance. (lEC 664 data indicates the lowest voltage for air breakdown is 360 volts peak; our test data indicates the lowest voltage is about 800 volts peak. At this time, I cannot explain this difference.)
A Further Look
Let’s take another look at what these data imply. Below the breakdown voltage, air is an insulator. Above the breakdown voltage, air is not an insulator. So, air is not always an insulator! What are the conditions, which must be fulfilled for air to be an insulator?
The answer is quite simple: The applied voltage must be less than the breakdown voltage. In mathematical form this can be expressed:
V (applied) < V (breakdown)
We can see from the graph that the breakdown of air, V (breakdown), seems to be a straight line. The equation of a straight line is of the form:
y = ax + b
where
y is the dependent variable,
a is the slope of the line,
b is the offset (value of y when x is zero), and
x is the independent variable.
The breakdown voltage for air, assuming a straight line, would be:
V (breakdown) = aD + b
where
a is the slope of the line in kilovolts/inch,
D is the distance, in inches, and
b is the offset, in kilovolts.
Using regression analysis (a function available in many handheld calculators), we can calculate the constants for the slope and the offset. The offset is about 0.8 kilovolt, and the slope is about 100 kilovolts per inch. So, the breakdown equation becomes:
V (breakdown) > 100D + b kilovolts
The conditions, which must be fulfilled for air to be an insulator, are:
V (applied) < V (breakdown)
Therefore,
V (applied) < 100D + 0.8 kilovolts
Let’s summarize where we are. Air, as an insulator, has some minimum voltage at which it will not break down, regardless of distance. Above that voltage, the breakdown voltage of air is directly proportional to the through-air distance between the two conductors, At any distance, if the applied voltage is less than the breakdown voltage for that distance, the air is an insulator. (Figure 2)
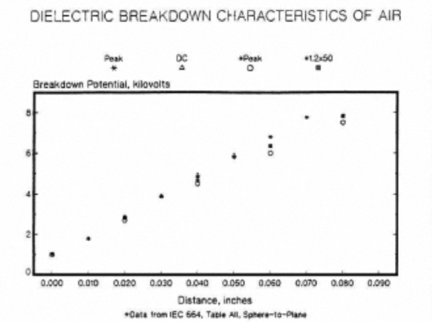
Figure 2
Conjecturing about Other Insulating Media
Intuitively, such characteristics should also apply to liquid and solid insulations. That is, for any insulation there is some minimum voltage at which it will not break down, regardless of distance, and, above that voltage, the breakdown voltage is directly proportional to the distance through the insulating medium. At any distance, if the applied voltage is less than the breakdown voltage for that distance, the material is an insulator.
The statement that there is some minimum voltage at which a material will not break down, no matter how thin, can be supported by the argument that if the solid insulating material is removed and replaced with air, then there is indeed a minimum voltage at which the air will not break down no matter how close the two electrodes. Therefore, a worst-case solid insulation cannot have insulation characteristics less than that of air.
Hypothesis: A material is an insulator if its breakdown voltage exceeds the applied voltage.
(Obviously, this is not a complete definition. but it is an absolutely necessary part of any definition.)
Conversely, a material is not an insulator if its breakdown voltage is less than the applied voltage.
Hypothesis: For any insulating material, whether solid, liquid, or gas, voltage breakdown is a straight line of the form:
V (breakdown) ~ aD + b
where
a is the slope of the line in kilovolts/inch,
D is the distance, in inches, and
b is the offset, in kilovolts.
Experience tells us that solid insulators are much “better” than air. “Better” is taken to mean that for the same distance, D, the breakdown voltage of solid insulation is very much greater than that of air. To satisfy the equation, “better” would mean that the value of “a,” in volts per unit distance, must be very much greater than that of air.
One of the current controversies is whether there is any difference in the breakdown voltage of solid insulation when the applied voltage is dc, ac, or the 1.2 x 50 µsec impulse. I›ll reserve discussion of this issue for another time.
Experimental Variables
In performing this experiment, there are a number of variables that must be controlled. The first is the shape of the electric field and the consequent uniformity of the equipotential lines. The second is the detection of the breakdown of air. The third is the measurement of voltage at the instant of breakdown.
When conduction occurs (a breakdown), the conduction will be along a line of force between the two conductors. Indeed, the breakdown will occur on the line where the electric force is greatest among all the lines of force that exist between the two conductors. The greatest electric force is on the shortest line of force.
In this experiment, it is essential that the electric force between the two conductors is uniform and that the equipotential lines between the two conductors are as uniformly spaced as possible. The electric field that produces uniformly spaced equipotential lines is described as a homogeneous field. The electric field is comprised of the lines of force between conductors. These lines of force emanate normal (at right angles) to the surfaces of the respective conductors. For each line of force, the potential between the two conductors divides uniformly along the line.
If we divide each line of force in half, and connect those points, we have an equipotential line, which represents one half the potential between the two conductors. Using this process; we can develop the pattern of equipotential lines between the conductors.
We can achieve a perfectly uniform electric field and, consequently, perfectly uniform equipotential lines if the two conductors are planes. But, the field at the edges of the planes would be rather non-uniform, and would therefore need to be accounted for in any experiment. To minimize the field distortion; we could gradually bend one plane away from the other. This would be done along the entire periphery of one plane; the resulting surface would be a plane with a spherical periphery (what you get at the instant a basketball bounces from the floor). The size of the plane is not at all critical, since the size of the electric field is not critical. Thus, the electric field resulting from a sphere in very close proximity to a plane approaches uniformity.
We used a 2-inch diameter steel sphere at distances from 0.01 inch to 0.10 inch from the plane. The distance ranged from 1% to 10% of the radius of the sphere. The scale illustration indicates the worst-case appearance of the two conductors.
The second variable is the detection of the breakdown of air. Fortunately, modern hi-pot testers have electronic trip mechanisms, which are uniform in tripping when an arc occurs. Any trip current is acceptable provided an arc truly occurs just before the trip. This is easy to confirm visually.
The third variable is the measurement of voltage at the time of trip. Here, a digital meter can be very helpful if the voltage is increased very slowly when approaching the breakdown.
Discrepancies with IEC 664
After performing the experiment and experiencing the repeatability, it seems appropriate to hypothesize why the differences between our data and the lEC data. When the lEC data is plotted point-by-point, the data agrees below 5 kV or so, and diverges seriously at 6.5 kv. This could be explained if the IEC had used a sphere where the ratio of distance to sphere radius was more than 10%. (The hi-pot tester available to me was limited to 6 kV rms and 6 kV dc, so we could not collect data as the ratio increased.) One hypothesis could he that nonlinearity can occur as the ratio increases.
At the lower voltages, the IEC data is not as precisely linear as our measurements. This could be explained by non-uniform observations of the breakdown or by poor control or measurement of the voltage. With the experience of performing the measurement, we found that these are critical to the uniformity and repeatability of the measurement.
There is still one more factor.
We chose steel as the material for the electrodes. Massive, thick steel. Whenever an arc occurs, the power dissipated in the arc can melt the metal at either end of the arc. But, with a good thermal conductor and lots of thermal mass, this is minimized. (In attempting to do the point-to-plane test, we burned up a hardened steel needle when the hi-pot failed to trip and let the arc continue for an undue amount of time.)
The Non-Uniform Electric Field
The other extreme is the perfectly non-uniform field and, consequently, non-uniformly distributed equipotential lines. Such a field is that resulting when the diameter of the sphere approaches zero.
A practical point is an extremely small sphere compared to the distance between the sphere and the plane. Since the lines of force must emanate at right angles to the surface of the small sphere, they are bent in the region of the small sphere and are therefore longer than the single line of force at the end of the sphere. Because the equipotential lines must be normal to the lines of force and equally spaced along the lines of force, the equipotential lines are severely bent near the small sphere. This bending of the equipotential lines increases the total force on any charged particle in the region of the point as compared to a homogeneous field. (Figure 3)

Figure 3
We also repeated the same test with a point-to-plane system. Immediately, we see significant differences. The first is a current indication well below breakdown. The second is lack of repeatability. The third is the slope of the line is about one fourth that of the sphere-to-plane.
Why these differences?
First, the highly bent equipotential lines lead to partial discharge at voltages very much less than the breakdown voltage. What happens is that the air actually breaks down in the region very near the point, but not across the entire gap. This is the same phenomenon as St. Elmo’s fire and the streamers that emanate from a Tesla coil, except on a much smaller scale.
Second, because the point has a very small thermal mass, the breakdown arc actually melts the steel at the point, and therefore changes the shape of the point. Thus, the next breakdown is at a slightly higher voltage because the point is less sharp and the equipotential lines in the region of the point are not as severely bent.
The effect of an imperfect electric field is to reduce the breakdown voltage at any given distance. The worst-case reduction seems to be about one fourth of the best-case.
Application
For a typical 120-volt rated product, the required clearance (UL and CSA) is 3/32 inch (0.094 inch). According to these data, and extrapolating the worst-case point-to-plane, 3/32-inch clearance should break down at no less than 3.47 kilovolts peak or de. The hi-pot potential specified by UL and CSA is either 1000 or 1500 volts RMS (1414 or 2121 volts peak, respectively). So, 3/ 32-inch clearance is more than adequate for the test voltage.
What clearance is necessary to withstand 2121 volts peak? Working backwards, we find that 0.041 inch (less than 1/2 millimeter) will withstand 1500 volts RMS! This is less than one half the distance required by UL and CSA standards!
We can only conclude that the requirements for clearances in various safety standards must be based on some parameter other than that of air as an insulator. ![]()
Acknowledgments
Impetus for this investigation was triggered by my friend and colleague, Jerry Blanz, who also participated in the experiment. Jerry is with Hewlett-Packard in Fort Collins, Colorado, and sits on CSA Subcommittee on No. 220, and Working Group 2 of IEC SC28A.
The required inequality of applied voltage and breakdown voltage was provided by another friend and colleague, Joe Neshiern, Joe is with Hewlett-Packard in Loveland, Colorado. Joe sits on CSA Subcommittee on No. 231, and on the US TAG to IEC 66E.
 |
Richard Nute is a product safety consultant engaged in safety design, safety manufacturing, safety certification, safety standards, and forensic investigations. Mr. Nute holds a B.S. in Physical Science from California State Polytechnic University in San Luis Obispo, California. He studied in the MBA curriculum at University of Oregon. He is a former Certified Fire and Explosions Investigator.Mr. Nute is a Life Senior Member of the IEEE, a charter member of the Product Safety Engineering Society (PSES), and a Director of the IEEE PSES Board of Directors. He was technical program chairman of the first 5 PSES annual Symposia and has been a technical presenter at every Symposium. Mr. Nute’s goal as an IEEE PSES Director is to change the product safety environment from being standards-driven to being engineering-driven; to enable the engineering community to design and manufacture a safe product without having to use a product safety standard; to establish safety engineering as a required course within the electrical engineering curricula. |
